Sublime
An inspiration engine for ideas
You and Your Research
Richard W. Hamming's lecture emphasizes the importance of pursuing significant research, setting high personal goals, and fostering confidence, creativity, and dedication to achieve excellence in one's career and contributions.
d37ugbyn3rpeym.cloudfront.netYou and Your Research - Richard Hamming (Bell Communications Research Colloquium, 1986)
Link
If you do not work on an important problem, it's unlikely you'll do important work. It's perfectly obvious. Great scientists have thought through, in a careful way, a number of important problems in their field, and they keep an eye on wondering how to attack them. (...) By important I mean guaranteed a Nobel Prize and any sum of money you want to ... See more
Richard Hamming • You and Your Research
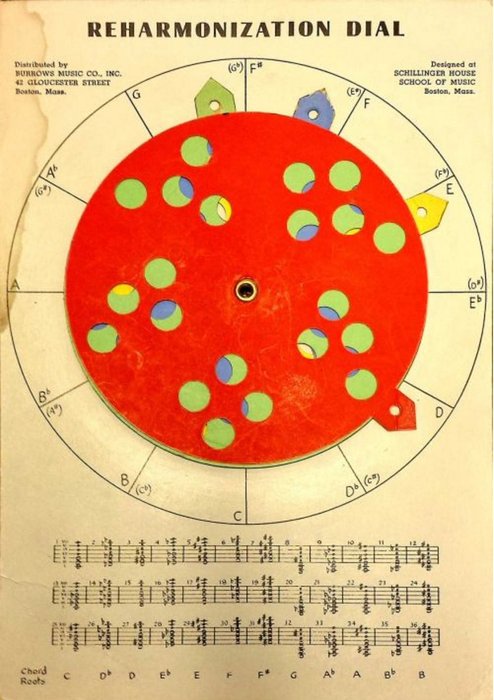
Joseph Schillinger’s Reharmonization Dial (1940) and a Bit More (The Hum Blog) : https://t.co/vwwKk7uG3w... See more

